Sugar Mountain

Last Saturday I got to go to the biannual meeting of the Macro-Finance Society. This is a great new effort spearheaded by outstanding young macro-finance researchers.

(The society is limited to people with PhDs after 1990, occasioning the title of this post, a reference to a song about a bar limited to people under 21, a reference you will not get unless your PhD was granted well before 1990.)

I can't blog all the great papers and discussions, so I'll pick one of particular interest, Itamar Drechsler, Alexi Savov, and Philipp Schnabl's "Model of Monetary Policy and Risk Premia"

This paper addresses a very important issue. The policy and commentary community keeps saying that the Federal Reserve has a big effect on risk premiums by its control of short-term rates. Low interest rates are said to spark a "reach for yield," and encourage investors, and too big to fail banks especially, to take on unwise risks. This story has become a central argument for hawkishness at the moment. The causal channel is just stated as fact. But one should not accept an argument just because one likes the policy result.

Nice story. Except there is about zero economic logic to it. The level of nominal interest rates and the risk premium are two totally different phenomena. Borrowing at 5% and making a risky investment at 8%, or borrowing at 1% and making a risky investment at 4% is exactly the same risk-reward tradeoff.



In equations, consider the basic first order condition for investment, \[ 0 = E \left[ \left( \frac{C_{t+1}}{C_t} \right)^{-\gamma} (R_{t+1}-R^f_t) \right] \] \[ 1 = E \left[ \beta \left( \frac{C_{t+1}}{C_t} \right)^{-\gamma} \right] R_t^f \] Risk aversion \(\gamma\) controls the risk premium in the first equation, and impatience \(\beta\) controls the risk free rate in the second equation. The level of risk free rates has nothing to do with the risk premium.

Yes, higher risk aversion or consumption volatility would increase precautionary saving and lower interest rates in the second equation, holding \(\beta\) fixed. But that is the "wrong" sign -- lower interest rates are associated with higher, not lower, risk premiums.

Worse, that "wrong" sign is what we see in the data. Risk premiums are high in the early part of recessions, when interest rates are low. Risk premiums are low in booms, when interest rates are high. OK, I'm a bit defensive because "by force of habit" with John Campbell was all about producing that correlation. But that is the pattern in the data. I made a graph above of the Federal Funds rate (blue) and the spread between BAA bonds and treasuries (green, right scale). You can see the risk premium higher just when rates fall at the early stage of every recession, and premiums low at the peaks of the booms, when rates are at their peaks.

So, if one has this belief about Fed policy, there must be some other effect driving a big negative correlation between risk premiums and rates, yet the Fed can cause premiums to go up or down a bit more by raising or lowering rates.

Every time I ask people -- policy types, central bankers, Fed staff, financial journalists -- about this widely held belief, I get basically psychological and institutional rather than economic answers.   Fund managers, insurance companies, pension funds, endowments, have fixed nominal rate of return targets. People have nominal illusions and don't think 8% with 1% short rates is a lot better than 10% with 9% short rates. Maybe. But basing monetary policy on the notion that all investors are total morons seems dicey. For one thing, the minute the Fed starts to exploit rules of thumb, smart investors change the rules of thumb. Segmented markets and institutional constraints are written in sand, not stone, and persist only as long as they are not too costly.

OK, enter  Drechsler, Savov, and Schnabl. They have a real, economic model of the phenomenon. That's great. We may disagree, but the only way to understand this issue is to write down a model, not to tell stories.

The model is long and hard, and I won't pretend I have it all right. I think I digest it down to one basic point. Banks had (past tense) to hold non-interest-bearing reserves against deposits. This is a source of nominal illusion. If banks have to hold some non-interest bearing cash for every investment they make, then the effective cost of funds is higher when the nominal rate is higher.  We are, in effect, mismeasuring \(R^f\) in my equation.

This makes a lot of sense. Except... Before 2007 non-interest-bearing reserves were really tiny, $50 billion dollars out of $9 trillion of bank credit. Quantitatively, the induced nominal illusion is small. Also, while it's fun to write models in which all funds must channel through intermediaries, there are lots of ways that money goes directly from savers to borrowers, like mortgage-backed securities, without paying the reserve tax. Banks aren't allowed to hold equities, so this channel can't work at all for the idea that low rates fuel stock "bubbles."

And now, reserves will pay interest.

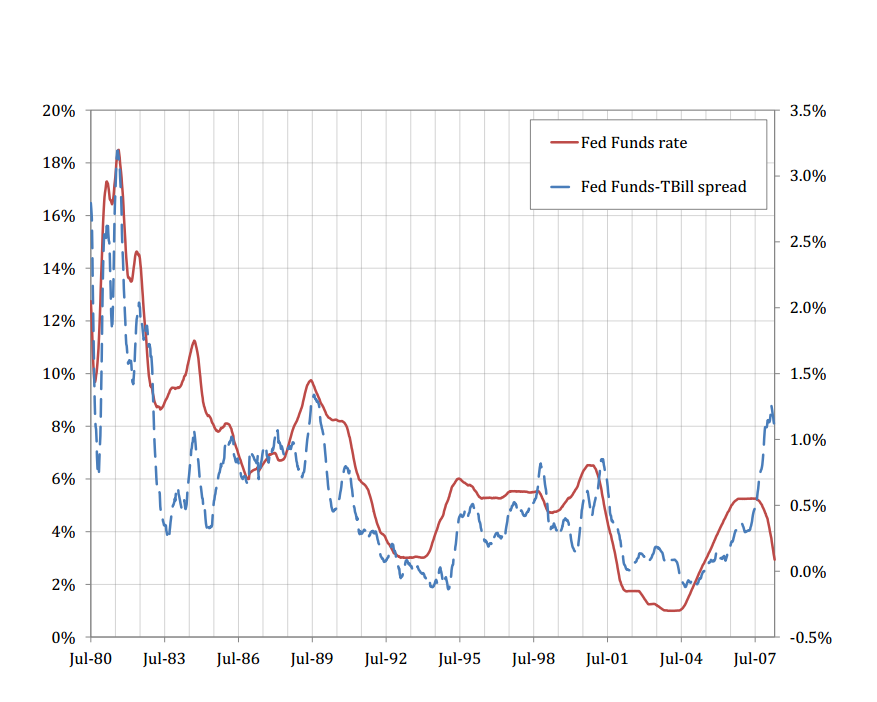
At the conference, Alexi disagreed with this interpretation. He showed the following graph:

Fed funds are typically higher than T bills, and the spread is higher when interest rates are higher. They interpret this quantity (p.3) as the "external finance spread." Fed funds represent a potential use of funds, and the shadow value of lending.  Alexi cited another mechanism too: "sticky" deposits generate a relationsip (at least temporary) between interest rate levels and real bank funding costs.   So by whatever mechanism, they say,  you can see that cost of funds vary with the level of interest rates.  In response to my sort of graph, yes, lots of other things push risk premiums around generating the negative correlation, but allowing the causal effect.

Read the paper for more. I have come to praise it not to criticize it. Real, solid, quantiative economic models are just what we need to have a serious discussion. This is a really important and unsolved question, which I will close by restating:

Does monetary policy, by controlling the level of short term rates, substantially affect risk premiums? If so, how?

Of course, maybe the answer is "it doesn't."